Transformations for repeated-measures t-test
Account for non-normal distributions when comparing two observations of a continuous outcome
The assumption of normality of difference scores is important when conducting repeated-measures t-tests. If the difference scores between two within-subjects observations are non-normal, there are really two viable options for answering the research question.
1. Researchers can identify any outliers (values that are more than 3.29 standard deviations away from the mean) for each observation of the outcome and make sure that the values were entered correctly. If there were, and the amount of outliers does not make up more than 10% of all observations, then researchers can delete the outliers in a "listwise" fashion. This means that the observation is deleted outright. This is the much less preferable to option two below.
1. Researchers can identify any outliers (values that are more than 3.29 standard deviations away from the mean) for each observation of the outcome and make sure that the values were entered correctly. If there were, and the amount of outliers does not make up more than 10% of all observations, then researchers can delete the outliers in a "listwise" fashion. This means that the observation is deleted outright. This is the much less preferable to option two below.
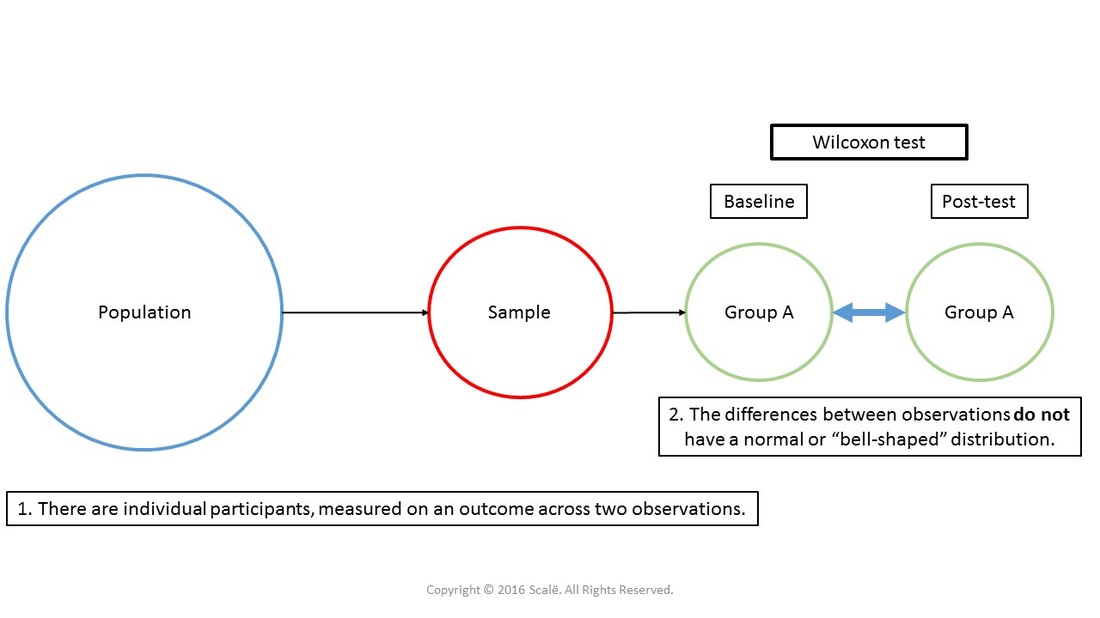
2. Researchers can run a non-parametric Wilcoxon test. Non-parametric tests are robust enough to handle violations of normality and still yield an interpretable p-value and effect. The figure below depicts the use of a Wilcoxon test when the assumption of normality of difference scores is violated for a repeated-measures t-test.
The steps for conducting a Wilcoxon test in SPSS
1. The data is entered in a within-subjects fashion.
2. Click Analyze.
3. Drag the cursor over the Nonparametric Tests drop-down menu.
4. Drag the cursor over the Legacy Dialogs drop-down menu.
5. Click 2 Related Samples.
6. Click on the first observation of the ordinal outcome.
7. Click on the arrow button to move the first observation of the outcome variable into the Test Pairs box under the Variable1 column.
8. Click on the second observation of the ordinal outcome.
9. Click on the arrow button to move the second observation of the outcome variable into the Test Pairs box under the Variable2 column.
10. Click OK.
2. Click Analyze.
3. Drag the cursor over the Nonparametric Tests drop-down menu.
4. Drag the cursor over the Legacy Dialogs drop-down menu.
5. Click 2 Related Samples.
6. Click on the first observation of the ordinal outcome.
7. Click on the arrow button to move the first observation of the outcome variable into the Test Pairs box under the Variable1 column.
8. Click on the second observation of the ordinal outcome.
9. Click on the arrow button to move the second observation of the outcome variable into the Test Pairs box under the Variable2 column.
10. Click OK.
The steps for interpreting the SPSS output for a Wilcoxon test
1. In the Test Statistics table, look at the p-value associated with Asymp. Sig. (2-tailed) row. This is the p-value that is interpreted.
If it is LESS THAN .05, then researchers have evidence of a statistically significant effect in the ordinal outcome across time or within-subjects.
If the p-value is MORE THAN .05, then researchers have evidence that there is NOT a statistically significant effect in the ordinal outcome across time or within-subjects.
If it is LESS THAN .05, then researchers have evidence of a statistically significant effect in the ordinal outcome across time or within-subjects.
If the p-value is MORE THAN .05, then researchers have evidence that there is NOT a statistically significant effect in the ordinal outcome across time or within-subjects.
Click on the Download Database and Download Data Dictionary buttons for a configured database and data dictionary for a Wilcoxon test.
Statistician For Hire
DO YOU NEED TO HIRE A STATISTICIAN?
Eric Heidel, Ph.D. will provide statistical consulting for your research study at $100/hour. Secure checkout is available with PayPal, Stripe, Venmo, and Zelle.
- Statistical Analysis
- Sample Size Calculations
- Diagnostic Testing and Epidemiological Calculations
- Psychometrics